what is the angle of the resultant force with respect to x-axis?

Table of Contents

• Introduction
• Definition
• Diagram
• Formula
• Examples
• Resultant force of multiple forces
• Calculator

Introduction

Imagine that a system of multiple forces, which have unlike directions and magnitudes, are interim in the same time on the same object (or point). The sum of all those forces is called the resultant force. The "sum" of all the forces tin also exist a difference, depending on the management of each force. Let'due south look at some simple examples for a improve understanding.

Image: Resultant force diagram – skydiver

If you lot think at a skydiver, when he (she) jumps out of a airplane, there are ii forces acting on him (her). The weight strength F_weight [N], which is pulling the skydiver towards the surface of the Earth, due to gravity, and the air drag force F_{air drag} [N], which is opposing to the motion of the skydiver through the air and tries to irksome him (her) down. As you can see, the weight forcefulness and the air drag force accept opposite directions, which means that the resultant R [North] will be the difference between them.

If we consider the positive management of the forces to be towards the surface of the then, the formula of the resultant strength is:

R = F_weight – F_{air drag} = 735 – 135 = 600 N

The result tells us that the skydiver will continue to fall towards the surface of the Earth because it is beingness pulled downwardly by the resultant force.

Go back

Definition

The resultant strength is the terminal force which acts on an object (body) subsequently combining via vector addition all the individual forces acting on the torso. With other words, the resultant force is a single force that has the same effect on the torso as 2 or more than forces acting in the same time.

Let'southward look at another case.

Paradigm: Resultant strength diagram – contrary directions of forces

On the point P there are two forces acting: the strength F_one [North] pulling to the right and the force F₂ [N] pulling to the left. Since the for F₁ is bigger than the force F₂, the resultant force R [North] will have the same direction as F₁ and the magnitude equal with the difference between F_ane and F₂.

R = F_ane – F₂ = 10 – 5 = 5 Due north

What happens if two forces act in the same direction?

Image: Resultant force diagram – same directions of forces

In this example both F₁ and F_ii forces pull the point to the correct. In this case the resultant force R will take the same management with F₁ or F_ii and its magnitude will be equal with the sum of the 2 forces.

R = F₁ + F₂ = 10 + 5 = 15 N

The resultant force unit of measurement of measurement is Newton [N] and the resultant force symbol is usually R.

Next, we are going to go into more details on the adding of the resultant forcefulness.

Go back

Diagram

In mechanics we deal with two types of quantities (variables): scalar and vector variables. Scalar variables have only magnitude, for example: length, mass, temperature, time. Vector variables have magnitude and management, for example: speed, force, torque. The direction of the vector is defined by the angles of the force witch each axis. The vector variables are ordinarily represented using bold symbols with arrows on summit.

Several forces can act on a body or bespeak, each force having different direction and magnitude. In engineering science the focus is on the resultant force acting on the body. The resultant of concurrent forces (acting in the same airplane) tin exist found using the parallelogram constabulary, the triangle dominion or the polygon rule.

Two or more forces are concurrent is their direction crosses through a common point. For case, ii concurrent forces F_ane and F₂ are acting on the aforementioned betoken P. In gild to notice their resultant R, we can apply either the parallelogram law or triangle rule.

Parallelogram constabulary	Triangle rule

The resultant strength is the vector sum between the components (if the equation is non displayed correctly RELOAD the page):

\[\overrightarrow{R} = \overrightarrow{F_1} + \overrightarrow{F_2}\]

If there are several forces acting on the same signal, we tin can utilize the polygon dominion to find their resultant.

\[\overrightarrow{R} = \overrightarrow{F_1} + \overrightarrow{F_2} + \overrightarrow{F_3} + \overrightarrow{F_4}\]

The resultant force can exist determined also for three-dimensional force systems, by using the polygon dominion.

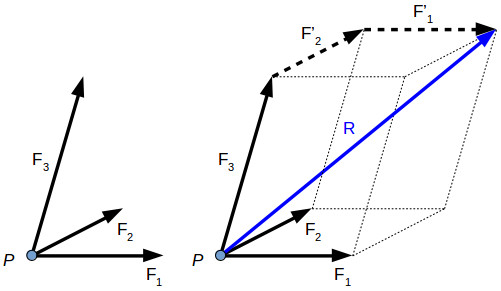
\[\overrightarrow{R} = \overrightarrow{F_1} + \overrightarrow{F_2} + \overrightarrow{F_3}\]

The parallelogram law, triangle rule and polygon dominion are geometric methods to find the force resultant. We can describe the forcefulness resultant but we don't know precisely its magnitude and direction.

In order to summate the magnitude and management of a resultant strength or to calculate the value of one force component or another, we tin can utilize the law of sines and the police force of cosines.

The diagonal of the parallelogram PBCA is the resultant force R, which forms 2 scalene triangles with the forces F₁ and F₂ .

Since the sum of all the angles within a triangle is 180°, nosotros tin can write γ role of α and β.

Get dorsum

Formula

The law of sines gives the human relationship between the forces and the angles:

\[\frac{F_1}{\text{sin}(\blastoff)} = \frac{F_2}{\text{sin}(\beta)} = \frac{R}{\text{sin}(180^{\circ} – \alpha – \beta)} \tag{2}\]

The law of cosines volition give the states the following human relationship:

\[R = \sqrt{F_1^2 + F_2^2 – two \cdot F_1 \cdot F_2 \cdot \text{cos}(180^{\circ} – \blastoff – \beta)} \tag{three}\]

The resultant forcefulness can likewise be calculated analytical, using force projections. Using the force projection method, we tin can calculate the magnitude and management angles of the resultant force.

In the paradigm higher up we have a resultant strength R and its projections on each axis:

F₁₀ – the project of R on the x-axis
F_y – the project of R on the y-centrality
F_z – the project of R on the z-axis
α – the angle between R and the ten-axis
β – the angle between R and the y-axis
γ – the angle between R and the z-axis

If in that location are several forces acting in the same point, we'll calculate the resultant of their projections on each axis:

\[ \begin{carve up}
F_x &= \sum_{i=1}^{n} F_{9}\\
F_y &= \sum_{i=1}^{northward} F_{iy}\\
F_z &= \sum_{i=i}^{n} F_{iz}
\end{split up} \]

where due north is the number of acting forces and F_x , F_y and F_z are the force resultants on each centrality.

The magnitude of the resultant force is:

\[R = \sqrt{F_x^two + F_y^ii + F_z^two} \tag{4}\]

Each axis resultant can exist expressed function of the resultant R:

F_x = R · cos(α)
F_y = R · cos(β)
F_z = R · cos(γ)

Replacing (4) in the equations in a higher place gives the angles with each axis (every bit trigonometric functions):

\[ \begin{split}
\text{cos}(\alpha) &= \frac{F_x}{\sqrt{F_x^ii + F_y^2 + F_z^ii}}\\
\text{cos}(\beta) &= \frac{F_y}{\sqrt{F_x^two + F_y^2 + F_z^two}}\\
\text{cos}(\gamma) &= \frac{F_z}{\sqrt{F_x^2 + F_y^2 + F_z^ii}}
\finish{dissever} \]

The forcefulness projection method can also be used for co-planar (x, y-axis) force resultant calculations.

Get dorsum

Examples

Example 1. Given the forces F₁ = 2.91 Due north, F₂ = 2.67 Northward, F_three = 2.47 North and F₄ = 2.23 N and the anglesα = 60° and β = 30°, calculate the resultant force R and its bendingγ with the x-axis.

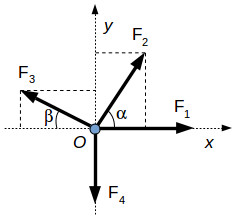
Pace 1. To get an idea on how the resultant force might await like, we tin can apply to polygon dominion.

Every bit yous tin run into, the magnitude of the resultant is nearly equal with that of the force F₃ . Also, the bending γ should be around the value of α. This geometrical solution is helpful because nosotros know what results nosotros should look from the analytical solution.

Stride two. Calculate the strength projections on each axis.

\[ \begin{split}
F_x &= F_1 + F_2 \cdot \text{cos}(\alpha) – F_3 \cdot \text{cos}(\beta) &= 2.11 \text{ N}\\
F_y &= F_2 \cdot \text{cos} \left ( \frac{\pi}{2} – \alpha \right ) + F_3 \cdot \text{cos} \left ( \frac{\pi}{2} – \beta \correct ) – F_4 &= 1.32 \text{ N}
\end{split} \]

Pace three. Summate the force resultant.

\[R = \sqrt{F_x^2 + F_y^2} = 2.48 \text{ N}\]

Step 4. Calculate the bending of the strength resultant with the x-axis.

\[\gamma = \text{arccos} \left( \frac{F_x}{R} \right ) \cdot \frac{180^{\circ}}{\pi} = 32^{\circ}\]

As expected, the analytic solution (forces projection) give the same results as the geometric solution (polygon rule).

Case 2. Given the forces F₁ = 6.12 N, F_two = 4.32 Due north, F_three = 1.84 N and their anglesα = xvi°,β = 22°,γ = 36°, calculate the force resultant R and its anglesα_R ,β_R ,γ_R  with the 10, y and z axis. The forces are diagonals on each side of a rectangular parallelepiped.

Stride ane. Calculate the force projections on each axis.

\[ \brainstorm{split}
F_x &= F_1 \cdot \text{cos}(\alpha) + F_2 \cdot \text{cos}(\beta) &= 9.89 \text{ N}\\
F_y &= F_1 \cdot \text{cos} \left ( \frac{\pi}{2} – \alpha \right ) + F_3 \cdot \text{cos} \left ( \frac{\pi}{ii} – \gamma \right ) &= ii.77 \text{ N}\\
F_z &= F_3 \cdot \text{cos}(\gamma) + F_2 \cdot \text{cos} \left ( \frac{\pi}{2} – \beta \right ) &= 3.11 \text{ North}
\end{split} \]

Step 2. Calculate the force resultant.

\[R = \sqrt{F_x^2 + F_y^2 + F_z^2} = x.7 \text{ N}\]

Step three. Summate the angle of the strength resultant with the x, y and z axis.

\[ \brainstorm{split}
\alpha_R &= \text{arccos} \left ( \frac{F_x}{R} \right ) \cdot \frac{180^{\circ}}{\pi} &= 22.viii^{\circ} \\
\beta_R &= \text{arccos} \left ( \frac{F_y}{R} \right ) \cdot \frac{180^{\circ}}{\pi} &= 75^{\circ} \\
\gamma_R &= \text{arccos} \left ( \frac{F_z}{R} \correct ) \cdot \frac{180^{\circ}}{\pi} &= 73.ii^{\circ}
\cease{split} \]

Go back

Resultant force of multiple forces

Now that nosotros understood how to calculate the resultant strength for an object, let'due south accept a more general arroyo. For two-dimensional issues, we can write down the general equations to summate the vertical and horizontal force components every bit:

• horizontal component:

\[F_{10} \text{ [Due north]} =\sum_{i=one}^{n}F_{i} \cdot \cos(\alpha_{i}) \tag{5}\]

• vertical component:

\[F_{y} \text{ [Due north]} =\sum_{i=1}^{n}F_{i} \cdot \sin(\alpha_{i}) \tag{half-dozen}\]

• resultant force:

\[R \text{ [N]} = \sqrt{F_x^two + F_y^2} \tag{7}\]

• angle of the resultant forcefulness with the horizontal axis

\[\gamma \text{ [} ^\circ \text{]}=\left\{\begin{matrix}
\arctan \left ( \frac{F_{y}}{F_{10}} \right ) \cdot \frac{180^\circ}{\pi}, \text{ if } F_{x}>0 \text{ and } F_{y}>0 \\
xc^\circ + \arctan \left ( \frac{\left |F_{x} \right |}{F_{y}} \right ) \cdot \frac{180^\circ}{\pi}, \text{ if } F_{x}<0 \text{ and } F_{y}>0 \\
180^\circ + \arctan \left ( \frac{\left |F_{y} \correct |}{\left |F_{10}\right |} \correct ) \cdot \frac{180^\circ}{\pi}, \text{ if } F_{x}<0 \text{ and } F_{y}<0\\
270^\circ + \arctan \left ( \frac{F_{x} }{\left |F_{y}\right |} \correct ) \cdot \frac{180^\circ}{\pi}, \text{ if } F_{ten}<0 \text{ and } F_{y}<0\\
\end{matrix}\right.\]

Example three. As case, allow's take the force system from Exercise 1 and calculate the resultant force and its angle with the horizontal axis (O-x).

For this method to work, all angles demand to exist referenced to the horizontal centrality, O-x.

The forces and angles are as follows:

• F₁ = 2.91 N, α_i = 0°
• F₂ = 2.67 N, α₂ = 60°
• F₃ = ii.47 Northward, α_iii = 150°
• F₄ = two.23 North, α_four = 270°

Stride ane. Summate the horizontal component of the resultant

\[F_{10} = 2.91 \cdot \cos (0) + 2.67 \cdot \cos (60) + 2.47 \cdot \cos (150) + 2.23 \cdot \cos (270) = 2.106 \text{ N}\]

Observation: If the adding is done on hand-held calculator of a software application, the argument of the cos() role must be given in radians, for example:

\[\cos \left ( 60 \cdot \frac{\pi}{180} \right )\]

Step 2. Calculate the vertical component of the resultant

\[F_{y} = 2.91 \cdot \sin (0) + ii.67 \cdot \sin (60) + ii.47 \cdot \sin (150) + 2.23 \cdot \sin (270) = 1.32 \text{ North}\]

Observation: If the calculation is done on hand-held computer of a software application, the argument of the sin() function must be given in radians, for example:

\[\sin \left ( sixty \cdot \frac{\pi}{180} \right )\]

Pace three. Summate the resultant force

\[R = \sqrt{two.106^{2} + 1.32^{2}} = ii.48 \text{ Due north}\]

Step 4. Summate the resultant force bending with the horizontal axis.

\[\gamma = \text{arctan} \left ( \frac{2.106}{2.48} \right ) \cdot \frac{180}{\pi} = 32.03^{\circ}\]

This method can be extended to any number of forces, every bit long as the force values and angles are known.

You tin can also check your results using the reckoner below.

Go back

Calculator

Fone [N]	Ftwo [Northward]	F3 [Northward]	F4 [N]	F5 [North]
α1 [°]	α2 [°]	α3 [°]	α4 [°]	α5 [°]
Horizontal force, F10 [Due north] =
Vertical force, Fy [N] =
Force angle, γ [°] =
Resultant force, R [N] =

Utilise the calculator higher up to calculate and evaluate dissimilar distribution of forces. By hovering the mouse pointer on the line forces, you can meet their coordinates which stand for the F_x [Northward] and F_y [North] components.

Don't forget to Like, Share and Subscribe!

zunigaloortambel.blogspot.com

Source: https://x-engineer.org/resultant-force/

Share this post